Making
Unfolding Humanity : ReTURN to Burning man (2023)
Each year, Burning Man Arts provides a limited number of grants aimed at partially financing artworks intended for placement on the open playa during the Burning Man event held in Black Rock City. Led by Diane Hoffoss (mathematics), this sculpture from 2018 was selected to receive an honorarium to help revise and install this artwork. The interactive sculpture, a steel dodecahedron covered in over 17,000 LEDs, highlights the tension between technology and humanity. One of the pentagonal walls of the sculpture unfolds under human power, inviting visitors to experience infinity reflections in the mirrored interior. The piece alludies to both Albrecht Dürer’s 500-year-old unsolved math problem on unfolding polyhedra and a possible shape of the universe. Ultimately, over a hundred students, faculty members and members of the community have worked on and revised the installation. This project was co-led with Austin Choi-Fitzpatrick (peace studies), Gordon Hoople (engineering), and Nate Padre (theatre). Photography by Frank Guthrie.
Fletcher Jones MatheMatics Studio (2020)
Mathematics has been built on Platonic notions, focusing on abstract ideas and theoretical structures. Yet we are physical beings who have bodies that manipulate and interact with the world around us. And so we ask: If mathematics has progressed so powerfully with the mind alone, how much more could be gained by considering the body as well? The centerpiece of a $1 million grant from the Fletcher Jones Foundation is for the creation of this mathematics research laboratory, designed to focus on embodied research mathematics. Based around the ideals of design thinking, artist workshops, and holy sites, governed by the philosophies of a shop (to make), a studio (to store), and a sanctuary (to favor the human senses over digital technology).
Mural : triangles and cubes TEssellation (2019)
This was a collaboration with my research student (Jordan Readyhough), focusing on art and architecture. It is a full-scale three-wall panel, installed in the new mathematics department entryway at the University of San Diego, located on the first floor of Serra Hall. Each panel of the wall shows a different view of a 3-cube: the largest panel (shown above) takes the net of a 4D cube, unfolds it into 3D, explodes it into 2D faces, and reduces the resulting parallelograms to tile a triangular grid. The shorter wall extends this grid and tiles it with a skewed Schlegel diagram of a 3D cube, followed by an orthogonal Schlegel diagram of the cube. The repetition and overlapping of the cubes within the grid breaks apart the elements of each cube, allowing for further abstraction of the diagrams. Upon entering the building, the short wall is primarily visible and the patterns begin smaller and simpler. However, as one approaches the wall or rounds the corner, each section of the mural gets progressively larger and bolder, drawing the viewer towards the entrance to the department.
Unfolding Humanity : Burning man (2018)
A two-ton metal, wood, and acrylic interactive sculpture, showcasing unsolved problems of mathematics, came to life in the middle of the Nevada desert. Rising 12 feet tall with an 18-foot wingspan, the unfolding dodecahedron was externally skinned with black panels containing 2240 acrylic windows, illuminated by over 16,000 LEDs which were programmed and driven by 20 controllers. The interior, large enough to hold 15 people, was fully lined with a massive mirror over each of the twelve pentagonal faces.
Our motivating question asks, what could it look like for vibrant and unsolved mathematics to be made embodied and physical, to engage the general public? And so, this sculpture deals with two unsolved questions: the unknown possibility of unfolding any convex polyhedra (motivated by 500-year-old works of Albrecht Dürer) and the shape of our universe (from WMAP cosmic microwave background radiation data and the Poincaré dodecahedral space).
With an estimated 6500 person hours invested and over $40,000 in grants and donations raised, the resulting artwork was displayed at Black Rock City, the desert location of Burning Man. This project was co-led with Diane Hoffoss (mathematics), a deeply collaborative effort with 80 volunteers and a faculty leadership team of Susie Babka (theology), Gordon Hoople (engineering), and Nate Padre (theatre). Photography by Gilles Bonugli Kali (instagram@gbk.style).
HEN bArn (2017)
This was a collaboration between a mathematician and an architect (Shannon Starkey), on the design and build of a chicken coop. It was motivated by a little known Frank Gehry hay barn design (1967) for Richard and Donna O’Neill on their ranch in San Juan Capistrano. Comprised of telephone poles and corrugated steel, the hay barn utilized similarly everyday materials as the residence, but without the latter’s formal complexity. Shannon's version is the Hen Barn, which appropriates and reimagines the hay barn as a functioning chicken coop. It features a twelve-foot chicken run above which the hen house floats to the left of the entry, accessed by a grooved ramp. The hen house features edge-to-edge ribbon windows and a rear hatch for retrieving the eggs.
The process was fast-tracked; drawings produced only just-in-time as needed. The mock-up, in this sense, produced, more than was produced by, the drawings. We were motivated to ask the question: how does the collaboration between math and architecture come into play? How does the real, built object re-evaluate the perfection of theoretical calculations and geometry?
williams college Course Catalog (2015)
The academic courses are the link between faculty and students. We present a redesign of the Williams College 2015-2016 course catalog, which removed a bureaucratic and administrative ordering and replaced it by highlighting what really matters: the courses. These appear in three distinctive formats:
a newspaper version, blending the classified ads of the old world and the twitter markings of the new.
a printed book, where the courses are not broken down by departments, but displayed in alpha order.
an online course explorer, providing a flexible, searchable style based on weightings, along with a visual calendar manager.
The cover of the printed book is shown above.
Cartography of tree space (2014)
In our enlightened world, the work of the mathematician and the visual artist are not only viewed as incompatible, but held in tension. The goal of this collaboration is to engage mathematics and the visual arts in a direct manner, with concrete outputs, that does not insult either field. In other words, new mathematical questions need to be formulated and new artworks need to be produced for the success of this venture. Our work is a true collaboration, with the mathematician (myself) involved in the drawings and the artist (Owen Schuh) involved in the mathematics.
The particular object of our study is a configuration space of phylogenetic trees. Each point in our space corresponds to a specific geometric, rooted tree with five leaves, where the internal edges of the tree are specified to be nonnegative numbers. From a global perspective, this tree space is made of 105 triangles, where three triangles glue along each edge. This results in 105 distinct edges, and 25 distinct vertices. This space of trees appears in numerous areas of mathematics, including algebraic topology, enumerative combinatorics, geometric group theory, and biological statistics.
The collaboration took place during an 18-month timeframe, from September 2013 until February 2015. Roughly, the first six months were spent in understanding the goals of the project and choosing a point of collaboration. The second six months were spent at coffee shops and studios, where we would meet and go through both mathematics and sketches. The final six months were focused on crafting extreme details and formulating a unifying vision for the project. Two painting of the triptych are shown above.
Shape of nature (2010)
While the mathematics involved in the study of shapes and nature is important to how we grasp and live in the world, it remains a mystery to many. Recruited and produced by The Great Courses, this 36-lecture course provides a pointed introduction to the language mathematicians use to study shapes and dimensions, covering some of the most beautiful ideas in geometry and topology, and aimed at the general public: read genetic data to better determine the relationships between species; closely study how proteins are built through the intricate process of folding; model and predict wind currents around the globe; map the seemingly random terrain of vast mountain ranges; develop facial recognition software for security systems; and design and improve the way that robots move and behave.
mural : Voronoi mathematics (2009)
The Williams College Mathematics and Statistics department is located on the second floor of the wonderful Bronfman Science Center. This second floor entrance occurs in a stairway, which has two bright red doors, with a large wall separating them. On this wall was a coat rack that had pegs to hold 25 coats. The goal was to transform this space into an entrance worthy of the department, to design and execute a site-specific piece of work. With ten students in my Mural course, we created this piece, with underpinnings dealing with:
a Voronoi diagram motivated from biological patterns.
two red tiles (hiding the light switch) that were Schlegel diagrams of the 3D associahedron polyhedron.
a four-coloring of the tiling, broken into two tree structures, with blue and yellow themes.
The mural is shown above, with two bright red doors on either side.
sculpture studio (2007)
What would happen if you explained five unsolved mathematical problems to five pairs of students, and asked them to visualize the concepts at hand, no holds barred? This was the premise behind the Geometric Modeling course. The results were spectacular, with sculptures made out of metal, wood, plexiglass, and computer graphics. The above photographs show two of the works.
The left piece deals with a metal skeletal framework of a 4D associahedron polytope, created by Eric Jonash and Sam Kapala. The vertices of this polytope correspond to all ways of triangulating the regular seven-gon, and the edges connect two such triangulations related by a flip. It was a long standing open problem (solved in 2012 by Lionel Pournin) which asks for the diameter of the associahedron for arbitrary dimensions.
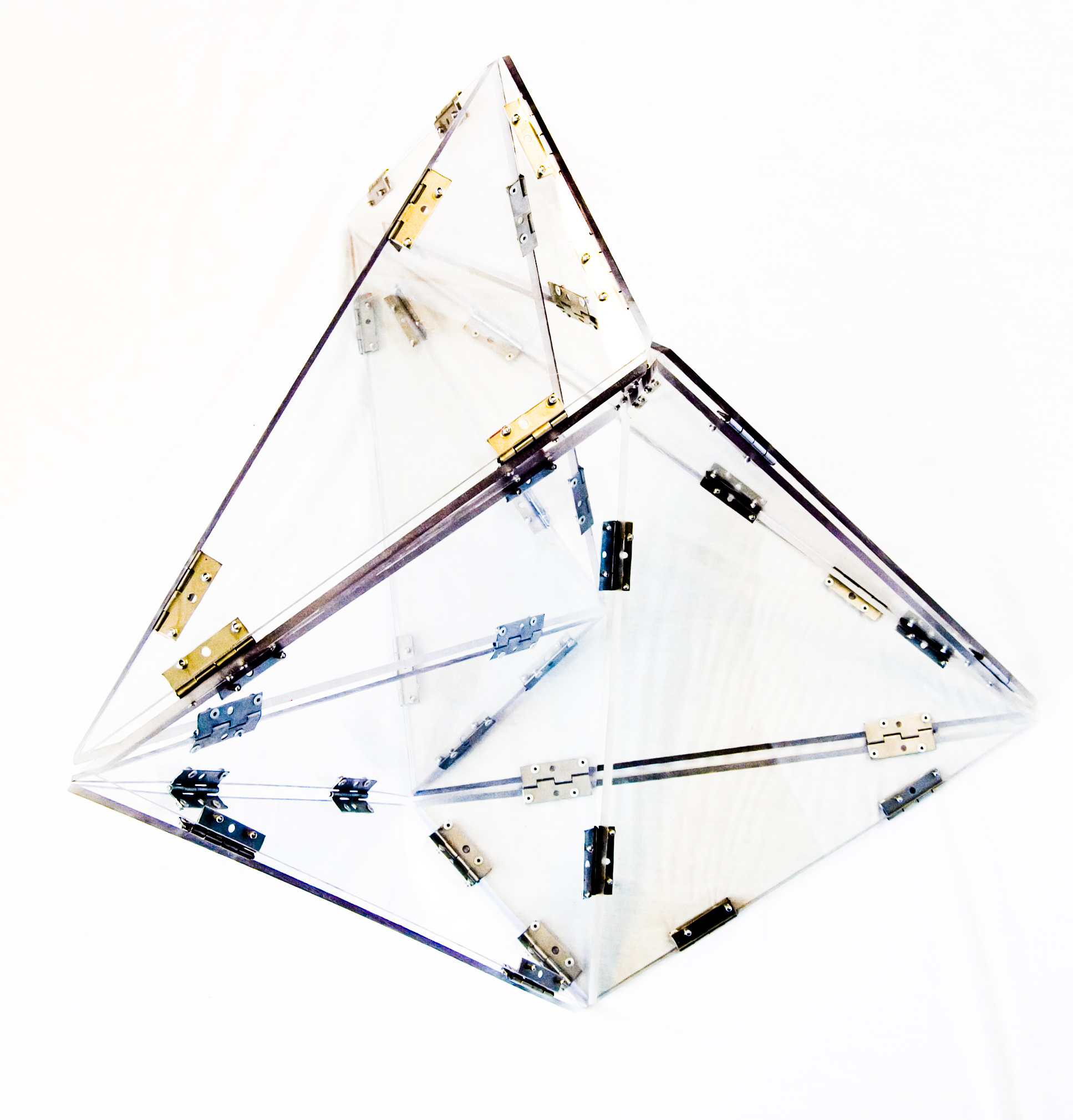
The right piece deals with the smallest flexible 3D polyhedron, made of plexiglass and piano hinges, created by Rohan Mehra and Norman Nicolson. Although the faces are rigid, the entire object flexes in a smooth, elegant manner, with a one-dimensional parameter of freedom. It is a stunning result of Sabitov that the volume of this (and any other) flexible 3D polyhedron remains constant during the deformation. It is an open problem whether the Dehn invariant alters during the flex.
photography
My mathematical research deals with structures of arbitrary dimensions. To understand work of such complexity, I am committed to bring a clear understanding for lower dimensional examples (such as dimensions two, three, and four). Since all of this is presented on flat paper (or monitor) to the reader, an incredible compression of data occurs. Thus, color, line width, and transparency are used to convey more information. Photography struggles with similar issues, capturing high dimensional information onto a flat canvas.